
The t-test gives the probability that the difference between the two means is caused by chance. Lastly, discussion on counted averages can be found on the Poisson help-page. Some more discussion on proportion averages can be found on the Binomial help-page. The certainty of these averages is directly related to the number of cases observed. Examples are the proportion of people suffering from complications comparing two different types of operation (number of complications on the number of operations), the proportion of a manufactured product damaged comparing two different methods of production (number damaged on the number manufactured). Also, you can compare ’proportion’ averages, basically a number divided by a larger number. The certainty with which these averages are measured are expressed in the standard deviation. You can compare ’continuous’ averages, they can be above or below one, examples are the difference in mean length or weight between two groups of people. It can be used to compare the difference in weight between two groups on a different diet, or to compare the proportion of patients suffering from complications after two different types of operations, or the number of traffic accidents on two busy junctions.
T-test concerns a number of procedures concerned with comparing two averages. Select the one sample option, other options and hit the calculate button.įor an explanation of the Pairwise t-test or the t-test for two correlated samples, consult the pairwise help page. Input either a population standard deviation in the first standard deviation box or a sample standard deviation in the bottom standard deviation box. Input a number of cases in the first number of cases box.

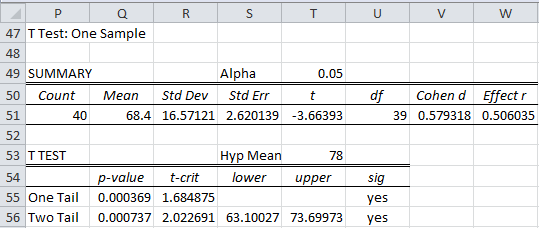
Input one expected normally distributed average in the top box and an observed average in the second box. To test for the significance of a difference between an average and an expected value. Input an expected proportion or number in the top box and an observed binomial proportion in the second box. To test for the significance of a difference between an expected and an observed proportion.

All the other boxes should have the value zero (’0’). Input an expected integer number in the top box and an observed number in the second box. To test for the significance of a difference between one observed Poisson count and an expected count. Select options and hit the calculate button.Ĭompare a single sample with the population For three or more averages use the oneway procedure. Input two observed real numbers in the top two boxes, two numbers of cases in the number of cases boxes and two standard deviations in the standard deviations boxes, so that there is a value in each box. To test for the significance of a difference between two normally distributed averages. For three or more percentages use the 2xr procedure. If you input a number it will be divided by the number of cases before the analysis to obtain a proportion. Input two observed proportions or two observed integer numbers in the top two boxes and two numbers of cases in the number of cases boxes. To test for the significance of a difference between two proportions or percentages.
Input two observed counts in the top two boxes. To test for the significance of a difference between two Poisson counts.


 0 kommentar(er)
0 kommentar(er)
